GRE Quantitative: Prime Factorization

As any Kaplan GRE Expert will tell you, the Quantitative section is a test of concepts, not calculations. It’s also a psychological test, designed to intimidate the rookie test-taker with big, scary-looking numbers.
Fortunately, there’s always a method to the GRE’s math madness and, consequently, a way of making those intimidating numbers easier to understand. That’s where the factor tree comes in—one of the most powerful weapons to add to your GRE math arsenal.

Factor tree basics

You may remember (fondly or not) making a factor tree in grammar school. Factor trees allow you to break a large number down into smaller, more manageable parts.
First, we should review the basics of how to find prime factors. We begin by using a garden variety factor tree whose fruit is composed of the essence of any positive, non-prime integer.
Suppose we want to find the prime factors of 60:

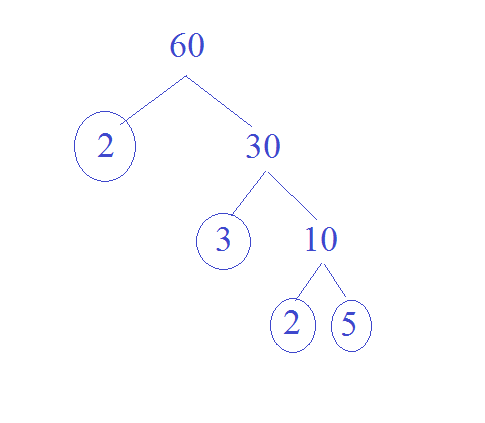
We can start with any two factors of 60 that are each less than 60, but together multiply to 60. Here, we use 2 and 30. Every time you hit a prime number, circle it, because that “branch” of the factor tree has reached its end. In this example, 2 is prime, so we circle it.
Then, we can break down 30 in the same way: We use 3 and 10, circling 3, since it’s prime. We then break 10 into 2 and 5, each of which is prime and gets circled as well.
This tells us a few things about the number 60. First, it tells us that its prime factors are 2, 3, and 5. Perhaps more importantly, it tells us that 60 can be expressed by multiplying all those numbers we circled a moment ago: 2 x 2 x 3 x 5.

Know your prime numbers

Prime numbers are the building blocks of any positive non-prime integer. In other words, any positive non-prime integer can be expressed as a bunch of prime numbers multiplied together.
It would certainly behoove any aspiring GRE champion to therefore memorize at least the first handful of prime numbers: 2, 3, 5, 7, 11, 13. You’ll notice that 2 is the smallest and the only even prime number. Remember, not all odd numbers after 2 are prime (you’ll notice that 9 is not prime because it is divisible by 3, for instance)—but after 2, all prime numbers are odd.

Making GRE math manageable

Finding prime factors can save you time and effort on the Quantitative section of the GRE if you know how to apply the concept. Whenever you feel like the GRE is trying to make you multiply or divide very large numbers—let’s use the very technical term “BUNs,” or Big Ugly Numbers—there’s a good chance that the shortcut involves prime factorization.
Imagine that the GRE gives you the following equation and asks you to solve for the value of b:

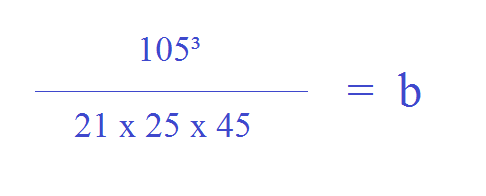
It would be time-consuming and inefficient on Test Day to determine what 105 x 105 x 105 is equal to, then find the actual value of 21 x 25 x 45, and then divide one BUN by another BUN.
The Kaplan-trained student thinks: “The GRE could’ve given me any numbers in the world, so there has to be some reason that they chose these.” In fact, this problem lends itself quite nicely to prime factorization, in which the math itself is relatively innocuous.

Prime factors are your friend

To solve the problem above, let’s start with the denominator. Using factor trees, we find out 21 = 3 x 7, 25 = 5 x 5, and 45 = 3 x 3 x 5.
Now let’s move to the numerator. The problem says 105³—so let’s just start with 105. Just by looking at 105, we know it’s divisible by 5, but 105 is 5 times what? Well, 5 x 20 would equal 100, in which case we’d need one more 5. So, 105 = 5 x 21, and 21 breaks down into 3 x 7 as we saw a moment ago. Therefore, 105 = 3 x 5 x 7. We have 105³, however. So, we must rewrite 3 x 5 x 7 a total of 3 times for the numerator. Now we have:

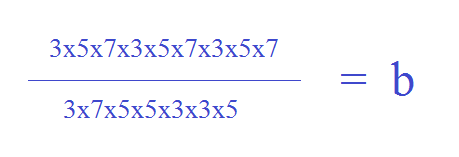
This becomes an exercise in what mathematicians call—to use even more technical terminology—”crossing stuff out.” Let’s cross off as many numbers on the top and bottom as we can, and see what is left standing. Cross out all of the 3’s, all of the 5’s, and just one 7 from the top and bottom—and voila: we are left simply with 7 x 7, or 49.
It involved some writing, but the math itself didn’t hurt too much now, did it?
Want to try out your factor tree skills? Sign up for a free GRE practice test and start building the confidence you need to boost your Quantitative score.