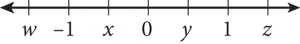PSAT Math: Exponents and Radicals

/in /by

We often turn to our calculators to solve difficult radical and exponent problems, especially in math-intensive classes. However, being too calculator dependent can cost you time and points on the PSAT. Further, on the PSAT, many radical and exponent problems are structured in such a way that your calculator can’t help you, even if it is allowed.

This chapter will review algebra and arithmetic rules that you may have learned at some point but likely haven’t used in a while. It will reacquaint you with the formulas and procedures you’ll need to simplify even the toughest expressions and equations on the PSAT.

We’ll start with exponents.

Exponents on the PSAT


Questions involving exponents often look intimidating, but when you know the rules governing them, you’ll see that there are plenty of shortcuts. First, it’s important to understand the anatomy of a term that has an exponent. This term is comprised of two pieces: a base and an exponent (also called a power). The base is the number in larger type and is the value being multiplied by itself. The exponent, written as a superscript, shows you how many times the base is being multiplied by itself.
Base ⇒ 34 ⇐ Exponent is the same as 3 × 3 × 3 × 3

The following table lists the rules you’ll need to handle any exponent question you’ll see on the PSAT.

Rule Example
When multiplying two terms with the same base, add the exponents.
ab × ac = a(b+c) → 42 × 43 = 42+3 = 45
When dividing two terms with the same base, subtract the exponents.
abac=a(b−c)→ 4342=4(3−2)=41
When raising a power to another power, multiply the exponents. (ab)c = a(bc) → (43)2 = 43×2 = 46; (2x 2)3 = 21×3 x2×3 = 8x6
When raising a product to a power, apply the power to all factors in the product.
(ab)c = ac × bc → (2m)3 = 23 × m3 = 8m3
Any term raised to the zero power equals 1. a0 = 1 → 40 = 1
A base raised to a negative exponent can be rewritten as the reciprocal raised to the positive of the original exponent.
a–b=1/ab; 1/a–b = ab →  4–2 = 1/42; 1/4–2=42

Different things happen to different kinds of numbers when they are raised to powers. Compare the locations and values of the variables and numbers on the following number line to the results in the table for a summary.

Quantity Even Exponent Result Odd Exponent Result Example
w positive, absolute value increases negative, absolute value increases (–5)2 = 25; (–5)3 = –125
–1 always 1 always –1 n/a
x positive, absolute value decreases negative, absolute value decreases
(–1/2)2 = 1/4; (–1/2)3 = –1/8
0 always 0 always 0 n/a
y positive, absolute value decreases positive, absolute value decreases
(1/4)2= 1/16; (1/4)3 = 1/64
1 always 1 always 1 n/a
z positive, absolute value increases positive, absolute value increases 32 = 9; 33 = 27

PSAT Math Practice Question: Exponents

Which of the following has the same value as  64 × 363 / 45?
  1. 39/2
  2. 310
  3. 22 × 39
  4. 312/22

Use the Kaplan Method for Math to solve this question, working through it step-by-step. The following table shows Kaplan’s strategic thinking on the left, along with suggested math scratchwork on the right.

Strategic Thinking Math Scratchwork
Step 1: Read the question, identifying and organizing important information as you go You’re asked to identify the expression that has the same value as the one presented; this means you need to simplify it.
Step 2: Choose the best strategy to answer the question As written, you can’t combine the bases or the exponents. However, 36 = 62, so rewrite the numerator to reflect this relationship. Then combine the bases in the numerator by adding the exponents. The bases are now being raised to the same power, 5, so rewrite the expression using a single exponent. Then simplify by dividing.   64 × 363 / 45 = (62)2 × 363 / 45 = 362 × 363 / 45   365/45 = (36/4)5 = 95
Step 3: Check that you answered the right question Although 95 is correct, it’s not one of the answer choices, so you’ll need to simplify even further. Rewrite 9 as 32 and then use exponent rules to simplify. The result is 310, which is (B).     95 = (32)5 =310

Radicals on the PSAT


A radical can be written using a fractional exponent. You can think of addition and subtraction (and multiplication and division) as opposites; similarly, raising a number to a power and taking the root of the number are another opposite pair.

Specifically, when you raise a term to the nth power, taking the nth root will return the original term. Consider for example 34 = 3 × 3 × 3 × 3 =81. If you take the fourth root of 81 (that is, determine the number that can be multiplied by itself four times to get 81), you will arrive at the original term: 4√81 = 4√(3×3×3×3) = 3 .
Radicals can be intimidating at first, but remembering the basic rules for radicals can make them much easier to tackle. The following table contains all the formulas you’ll need to know to achieve “radical” success on the PSAT.
Rule Example
When a fraction is under a radical, you can rewrite it using two radicals: one containing the numerator and the other containing the denominator.
√(a/b) = √a / √b → √(4/9) = √4/√9 = 2/3
Two factors under a single radical can be rewritten as separate radicals multiplied together.
√(ab) = √a × √b → √75 = √25 × √3 = 5√3
A radical can be written using a fractional exponent.
√a= a(1/2),3√a = a(1/3) → √289 = 289(1/2)

a(b/c) = c√ab → 5(2/3)→3√52
When a number is squared, the original number can be positive or negative, but the square root of a number can only be positive. If a2 = 81, then a = ±9, BUT √81 = 9 only.

It is not considered proper notation to leave a radical in the denominator of a fraction. However, it’s sometimes better to keep them through intermediate steps to make the math easier (and sometimes the radical is eliminated along the way). Once all manipulations are complete, the denominator can be rationalized to remove a remaining radical by multiplying both the numerator and denominator by that same radical.

1. Original Fraction 2. Rationalization 3. Intermediate Math 4. Resulting Fraction
x/√5 x/√5 × √5/√5
x√5/√(5×5) = x√5/√25 = x√5/5
 x√5/5
14/√(x2+2)
14/√(x2+2) × √(x2+2)/√(x2+2)
14√(x2+2) / √(x2+2)(x2+2) = 14√(x2+2)/√(x2+2)2
 14√(x2+2)/x2+2

Sometimes, you’ll have an expression such as 2 + √5 in the denominator. To rationalize this, multiply by its conjugate, which is found by negating the second term; in this case, the conjugate is 2 − √5  . As a general rule of thumb, you are not likely to see a radical in the denominator of the answer choices on the PSAT, so you’ll need to be comfortable with rationalizing expressions that contain radicals.

Note

When you rationalize a denominator, you are not changing the value of the expression; you’re only changing the expression’s appearance. This is because the numerator and the denominator of the fraction that you multiply by are the same, which means you’re simply multiplying by 1.

Ready to take on a test-like question that involves radicals? Take a look at the following:

PSAT Math Practice Question: Radicals

2. If 4√x − 8 =  –2 , what is the value of x + 4 ?
  1.    629
  2. 1,300
  3. 1,628
  4. 2,405

Work through the Kaplan Method for Math step-by-step to solve this question. The following table shows Kaplan’s strategic thinking on the left, along with suggested math scratchwork on the right.

Strategic Thinking Math Scratchwork
Step 1: Read the question, identifying and organizing important information as you go All you need to do is solve for x.
Step 2: Choose the best strategy to answer the question What should you do first? Solving a radical equation is similar to solving a linear equation, so start by isolating the variable term on one side. What operation will remove the root from the left side of the equation? To undo the radical, apply the exponent that corresponds to the root (4 in this case) to each side.     4√x − 8 = –2

4√x=6

(4√x)4 = 64

x = 64

x=1,296
Step 3: Check that you answered the right question You’ve found x, so add 4 and you’ll be done. The correct answer is (B).   x + 4 = 1,300